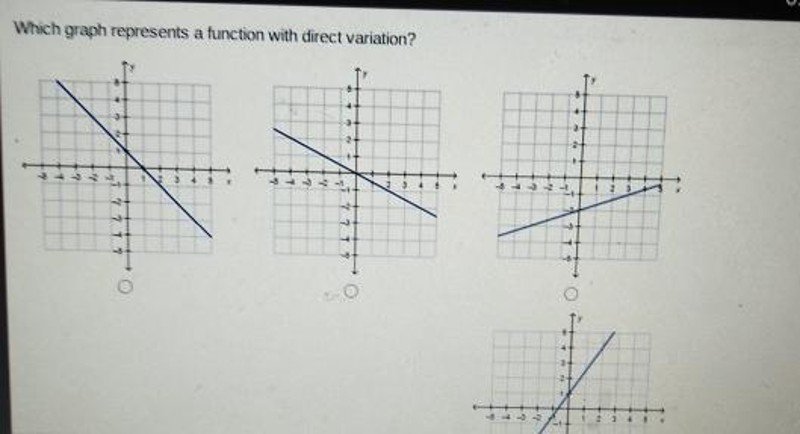
which graph represents a function with direct variation
Types of Graphs
A function with direct variation has a linear relationship. A straight line that passes through the origin (0,0) represents this relationship. The equation for a function of this type is y = kx. If the slope is zero, the graph is a straight line. If the slope is positive, it is a quadratic function. Alternatively, a direct-variation function may be a cubic, quartic, or radical.
Direct and Inverse Variation Functions
A direct-variation function is the function whose value increases with time. If you want to graph it, you will plot a straight line through the origin. An inverse-variation function will have a curve that approaches the x-axis as x increases. Which graph does the function in question have? Which one represents a function with direct variation? How do you determine whether a graph represents a function?
Graph changes on the base of Conditions
If the value of a variable changes with time, the graph will show this variation. Y/x changes over time. In direct-variation, a graph shows the change in value as a function of x. The slope is k, and the curve is directly related to y. If the inverse-variation graph shows an increase in y, the graph of a variable equals x+k.
Straight Line Graph
A direct-variation graph passes through the origin. This graph is a straight line with a proportional relationship between x and y. The slope of the line is the k-value of the direct-variation function. Y is the value of k in the x-y equation, and x increases. The inverse-variation graph is a curve that moves away from the x-axis, and k is equal to x.
First Analyze the type of Variation
In a graph analysis problem, the goal is to determine whether the graph represents a function with direct variation or an inverse-variation. A direct-variation graph will be a straight line that passes through the origin, whereas an inverse-variation will have a curve that approaches the x-axis as x increases. This is not an easy problem to solve. Therefore, you must first understand how to analyze a direct-variation graph.
Examples
The graph of a function with direct variation is a straight line that passes through the origin. It will have a constant value of k, and the x-axis will be an arbitrary point. Inverse-variation graphs are the inverse of a direct-variation graph. This is not a graph that is a straight line. For example, a curve with the slope of x+k would be a line that passes through the origin.
Conclusion
A graph representing a function with direct variation is a straight line that passes through the origin. Inverse-variation graphs are slanted downward from left to right. By dividing x and y values, you can determine which is the correct graph to represent a function with direct variation. These diagrams may be difficult to interpret. If you don’t understand them, you can read the equation and the data in the paper.
Visit Digital Global Times for more quality articles.